Measurement Accuracy Enhancement of Distributed Brillouin Sensors Based on Gain and Losses

A novel method for Brillouin optical time-domain analyzers exploiting the superposition of gain and two losses without four-wave mixing has been proposed. Doubled frequency accuracy enhancement has been demonstrated.

For the Brillouin distributed fiber sensor, the measurement of the Brillouin frequency shift (BFS) is highly dependent on the retrieving of the Brillouin gain spectrum (BGS). However, due to the fiber loss the decreasing probe signal power leads to an increase of the BFS measurement error. In this paper, a novel method is proposed to enhance the BFS accuracy by applying Brillouin gain and two losses. In comparison with the conventional method, doubled measurement accuracy has been demonstrated in the simulation.

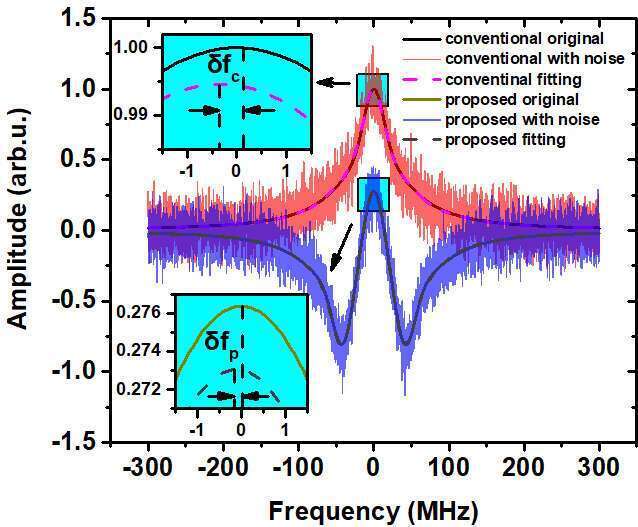Fig.1

Fig.1 shows the simulation steps. First, a normalized Voigt function profile V(f) (black solid line in Fig.1) is set as the BGS of a conventional sensor with its full-width at half maximum labelled as FWHM. The BGS of our proposed sensor can be expressed as a superimposed Voigt function, i.e. V(f)+mV(f+d*FWHM)+mV(f-d*FWHM), where m is the ratio between the maximum gain of a conventional sensor and the maximum loss, d is the ratio between frequency shift of the loss center to the gain center and the FWHM. For a given parameter pair (m, d), the same level of noise is added upon both BGS profiles. These noise added profiles are fitted with a (superimposed) Voigt function and the offsets of the peak frequency (BFS) due to the noise are recorded as δf_ci (or δf_pi) after the i-th fitting, as depicted in the insets of Fig.1. The fitting process is repeated by n = 300 times and the ratio of the standard deviation of the n frequency offsets is calculated for both cases, i.e.:

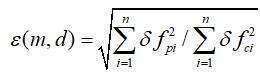
The distribution of ε in Fig.2 indicates that our proposed method has a better measurement accuracy with the largest improvement (2.2 times) achieved when m = 1 and d = 0.76.

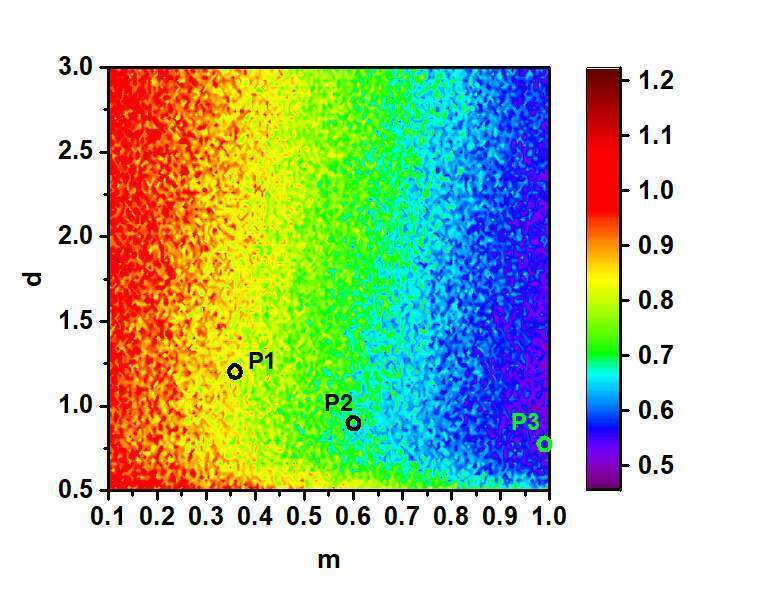Fig.2

The BFS measurement error along the fiber is also simulated. Two random (m, d) pairs (P1 and P2 in Fig.2) together with the best case (P3 in Fig.2) are chosen as examples in comparison to the conventional sensor. As indicated in Fig.3, the BFS measurement error in all cases follow the exponential fitting very well and the measurement accuracy enhancement of our proposed sensor shows also agreement with the result in Fig.2.

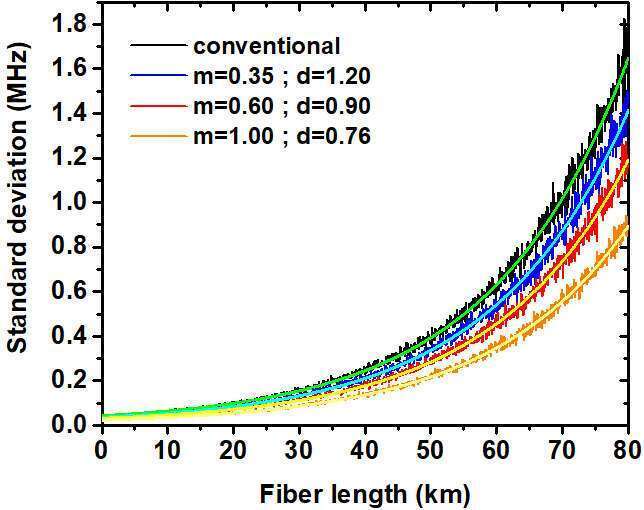Fig.3

In conclusion, a novel method of superimposing Brillouin gain and losses without four wave mixing and modulation instability is applied on Brillouin sensing. In comparison with the conventional sensor, more than two times of BFS measurement accuracy has been demonstrated. Under the same error tolerance, a longer sensing range can be expected.

cheng.feng@ihf.tu-bs.de