Abstract:
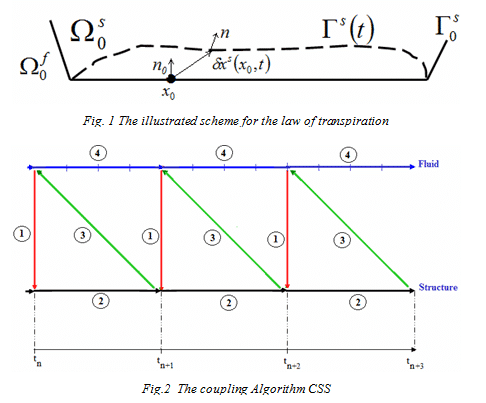
The aim of this study is to develop a new approach for the aeroelastic stability analysis of a flexible over-expanded rocket nozzle using numerical fluid-structure coupling via the transpiration method. The method consists of a weak coupling between two numerical codes; one is dedicated to the Computational Structural Dynamics (CSD) and the other to the Computational Fluid Dynamics (CFD). Indeed, given the context of the study, which is limited to the analysis of linear stability relative to the small displacements, here, the ALE (Arbitrary Lagrangian-Eulerian) formalism, based on the mesh dynamic aspect, widely discussed in many previous studies loses its attractiveness in favour of the transpiration method. In fact, the choice of the transpiration technique to consider the coupling at the fluid-structure interface is justified by its simple implementation and its low CPU time computation compared to those of the ALE method. It only requires a simple reformulation of the boundary conditions at the solid wall. This advantage allows immediate use without major changes in the fluid code. This method is inspired from the work of Fernández Varela who applied this technique to study aerodynamic wing stabilities (Fig.1). Subsequently, the results issued from the coupled computation are compared with those of the aeroelastic stability models developed in previous works.
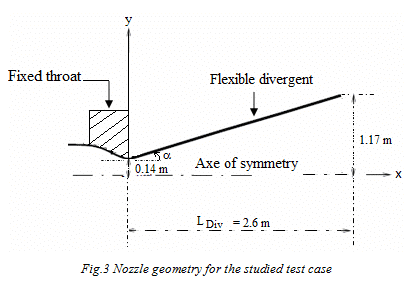
The fluid dynamic solver used for solving the averaged Navier-Stokes equations is the NSC2KE code developed at INRIA by Mohammadi. The code uses a combination of the finite volume and finite element methods to simulate perfect or viscous flows around or inside 2D or axisymmetric configurations on unstructured meshes. The structure dynamic solver is based on the implicit Newmark scheme to describe the temporal evolution of the structure displacements. For the coupling technique and for temporal integration, unlike to the code of structure, the NSC2KE fluid code is based on an explicit scheme in which the fluid time step is conditioned by the CFL stability condition. This difficulty leads to a time shift between the two codes, which can be overcome by using the iterative scheme CSS (Conventional Serial Staggered) (Fig.2). The implementation of the coupling procedure is straight forward; it consists in first step to obtain the converged aerodynamic field of the supersonic nozzle flow, initially assumed rigid for a given NPR via a CFD calculation. In the second step, the structure is disturbed according to one of its own modes, generally the first mode. This step provides the initial effort to transmit to structure code and it is from this moment that the coupling establishes between the two codes.
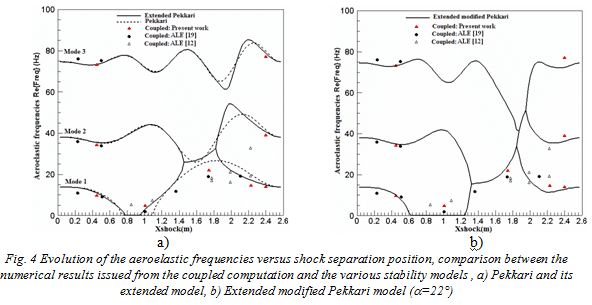
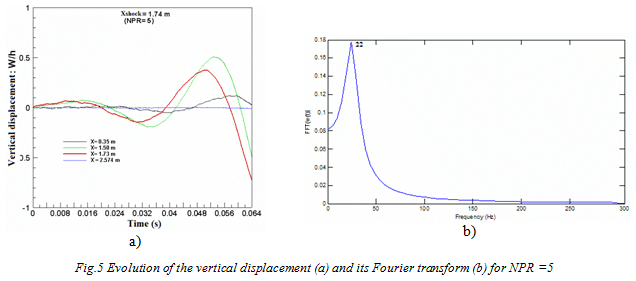
In this study and in order to compare the results issued from the aeroelastic stability models to these of the numerical coupling, 2D nozzle geometry has been chosen with fixed nodes at throat and at the exit of the nozzle divergent (Fig.3). Figure 4 shows the evolution of the real part of the aeroelastic frequency for the first three modes versus shock wave position. As we can see, the results predicted by the numerical coupling are in general in good agreement with those issued from the models predictions. In addition, the coupled computation indicates that the temporal evolution of the normal structure displacement and their Fourier transform, adopt the same behaviour in the region of flutter instability as shown in figure 5. This evolution is harmonic having an increasing amplitude, characterizing the phenomenon of dynamic instability or flutter. This type of instability may leads to the structure failure and represents a major issue in industrial context. Despite the limitations of coupling based on the method of transpiration, the results show that a linear approach for the small displacements is usually sufficient for the dynamic instabilities prediction. Beyond the linear range for large displacements and rotations, the method of transpiration becomes inoperative. In this case, the use of techniques allowing to take into account the dynamic mesh effect, the ALE method, for example, is unavoidable. The final paper will give a more detailed description of the numerical coupling and will contain further comparison between the present method and these of the approach based on the aeroelastic stability models.