Over the last decade, a renewed interest in manned flights to the Moon and Mars has brought back attention to the field of hypersonic aerothermodynamics. The recent successful examples of the Chang`e 3 mission to the Moon by the China National Space Administration (CNSA), and the Mars Orbiter Mission (MOM) by the Indian Space Research Organization (ISRO) even inspire the engineers. Currently, several private companies are designing hypersonic vehicles (ZEHST by EADS and Dragon by SpaceX) that, hopefully, will become the next generation space vehicles.
When the space vehicle enters the atmosphere at an orbital speed, a detached bow shock forms in front of its blunt nose and the temperature behind the shock, especially in the stagnation region, can reach high levels, due to the conversion of kinetic energy into thermal energy by strong shock compression. The important gas molecule dissociation and other real gas effects of hypersonic flows will occur. Consequently, the air can no longer be treated as a perfect gas and the dissociative non-equilibrium phenomenon complicates the flow physics.
Although the nose of a space vehicle is not completely a sphere, many previous theoretical, experimental and numerical researches concentrated on the hypersonic flows over spheres for model simplification. Many flow physics and flow features can be extracted from such spherical nose flows and some significant analytical solutions can be derived. Due to the extreme flow conditions, experimental approaches to study the hypervelocity flows are difficult in general, not to mention expensive. Therefore, to thoroughly understand the hypersonic dissociative non-equilibrium flows, it is essential to develop a reliable, efficient and accurate numerical method.
In this work, inviscid and viscous hypersonic dissociating flows over spheres are studied using the space-time conservation element and solution element (CE/SE) method proposed by Chang and To1 , based on meshes with triangular and quadrilateral elements2. Several cases of nitrogen, air, carbon dioxide flows, are provided to demonstrate the accuracy and reliability of the method regarding inviscid and viscous non-equilibrium flows. Since its inception in 1991, the CE/SE method, using the unique approach of enforcing flux conservation in both space and time with extensive physics considerations, has been used to obtain highly accurate numerical solutions for flow problems involving shocks, contact discontinuities, etc.
For the inviscid flows over a sphere, special attention is placed on the reliability of the code to predict the two important flow characteristics—shapes of the bow shocks and the stand-off distances, Δ. The results provided by the CE/SE code were compared against the theory and experiments performed by Wen & Hornung3. Good agreement among experimental, theoretical and numerical results for CO2 flows is observed in Table 1. The numerical shapes of the entire bow shocks for different gases are also very close to the experimental results. A typical example of CO2 flow is shown in Fig. 1.
Table 1. Comparison among experimental, theoretical and numerical results for CO2 flows
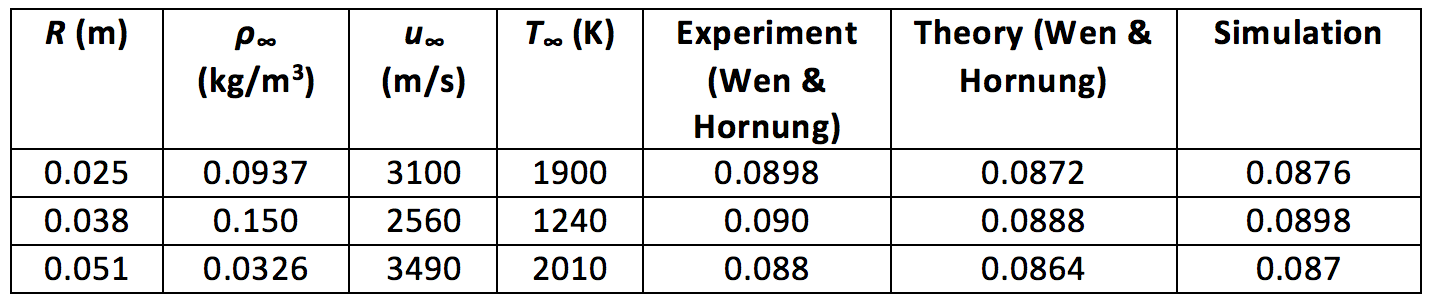
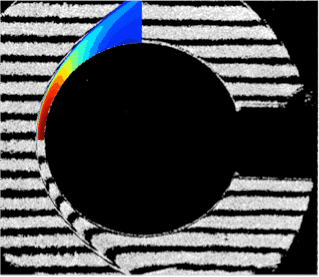
Figure 1. Comparison of numerical and experimental bow shock shapes for a CO2 flow case around a sphere of R=5.1 cm in Table 1.
For viscid cases, the reflection of a normal shock wave from the end wall of a shock tube has been simulated to validate the code and to investigate the unsteady, viscous interaction aspects of the shock bifurcation. In this test case, the shock bifurcation is simulated based on experimental conditions in Matsuo et al4. To save computational resources, only was the last 0.15m from the end wall and half a domain of 0.02m simulated. The results provided by the CE/SE code were then compared against the experimental results of Matsuo et al. and the numerical results of Weber et al5. Figure 2 shows the triple point trajectories from the CE/SE simulations with the adiabatic wall boundary condition, the calculations from Weber et al. and the experimental values of Matsuo et al. As seen, the CE/SE results follow a trend that predicts the triple-point trajectory similarly to the results of the reference calculations and the experimental results. However, the calculations predict an equilibrium height 20% higher that the experimental one, being similar with the isothermal simulations of Weber et al. Two possible reasons may cause this discrepancy. The first one is a growth of the interacted zone caused by the lower density found in an adiabatic wall boundary layer and the second reason is the lack of a turbulence model in the simulation. Therefore, the viscous layer contains less energy, yielding a large displacement. A future analysis applying the isothermal wall boundary condition will be performed and presented.

Figure 2. Comparison of the variation of the bifurcated foot height with distance from the end wall. Incident Mach 2.6.
Furthermore, results of viscous air, CO2 and nitrogen flows over spheres will be presented in detail in the conference.
1 S. C. Chang and W. M. To, A New Numerical Framework for Solving Conservation Laws - The Method of Space-Time Conservation Element and Solution Element, NASA, 1991.
2 H. Shen, C.Y. Wen, K.X. Li, and D.L. Zhang, Robust High-Order Space-Time Conservative Schemes for Solving Conservation Laws on Hybrid Meshes, Journal of Computational Physics, rebuttal, 2014.
3 C.Y. Wen and H. G. Hornung, “Non-equilibrium dissociating flow over spheres,” J. Fluid Mech., vol. 299, no. 1, pp. 389–405, 1995.
4 K. Matsuo, S. Kawagoe, and K. Kage. The interaction of a reflected shock wave with boundary layer in a shock tube, Bull JSME 17, 1039 (1974).
5 Y. S. Weber, E. S. Oran, J. P. Boris, and J. D. Anderson, The numerical simulation of shock bifurcation near the end wall of a shock tube, Phys. Fluids, vol. 7, no. 10, p. 2475, 1995.

