The area of shock focusing has been studied for a long time period, and its applications have been expanded to diverse areas such as industrial and civil engineering, biomedical, anti-terrorist and aerospace industry. It is of interest to understand and predict how interaction and coalescence between multiple blast waves can help to direct energy towards a target while simultaneously reduce collateral damage.
A finite amount of energy was assumed to be released instantaneously. Self-similar solutions, which were developed by Taylor [1] for a strong shock assumption, were implemented as initial conditions. A second order Godunov scheme was employed to solve the Euler equations of gas dynamics of the blast wave propagation. A Gaussian function was implemented as a source term and added to the Euler equation to control the delayed initiation time of munitions.
In this work, multiple munitions were placed in a concentrical patterns around a target. For all simulations, the total energy summed up over all munitions is kept constant. Results have been compared for a simulations with varying (i) number of munitions, (ii) radial distance to target, (iii)adiabatic index, and (iv) individual munition delay times.
Results show that a polygonal converging shock is formed by interaction of multiple shocks during their propagation towards the target, see Figure 1. The polygonal-shaped shock reconfigures and accelerates during the whole focusing process. In order to further study this phenomenon, a numerical approach based on Whitham’s theory [2] of geometrical shock dynamics (GSD) has been implemented. This is an approximate theory of shock dynamics without taking the flow field behind the shock into account.
The effect of adiabatic index was investigated by changing the ratio of specific heats of the gas from γ= 1.4 to γ= 1.1. The results show that the general trend is conserved, i.e.it is beneficial touse multiple munitions instead of a single, albeit more powerful one, see Figure 2. However, when comparing the resulting peak pressure at the target for 1, 3, 5 and 10 simultaneous munitions for the two types of gases, differences are observed. The peak pressure is lower, higher, lower and higher for the 1, 3, 5 and 10 cases, respectively. For a single munition with the same initial conditions except a change in the shock velocity for γ= 1.1 is lower than γ= 1.4. Therefore, in the case with a single munition, the blast wave generated for γ= 1.1 took longer time to reach the target than for the case of γ= 1.4, resulting in higher energy dissipation during the propagation and lower peak pressure at the target. For cases with 3, 5, and 10 munitions, there are several reasons why the results differ. In the case of γ= 1.1, a higher density is obtained behind the blast wave, which means mass is more concentrated during the shock interaction process. Second, a lower shock velocity is observed in the γ= 1.1 case compared to the γ= 1.4 case, which means longer time is available for shock-shock interactions and shock reconfigurations during the focusing process. Third, pressure dissipates faster in the case of γ= 1.1 than compared to the case of γ= 1.4.
Optimization is also investigated to further analyze the shock focusing effects based on initial location of individual munitions and different geometry of targets.
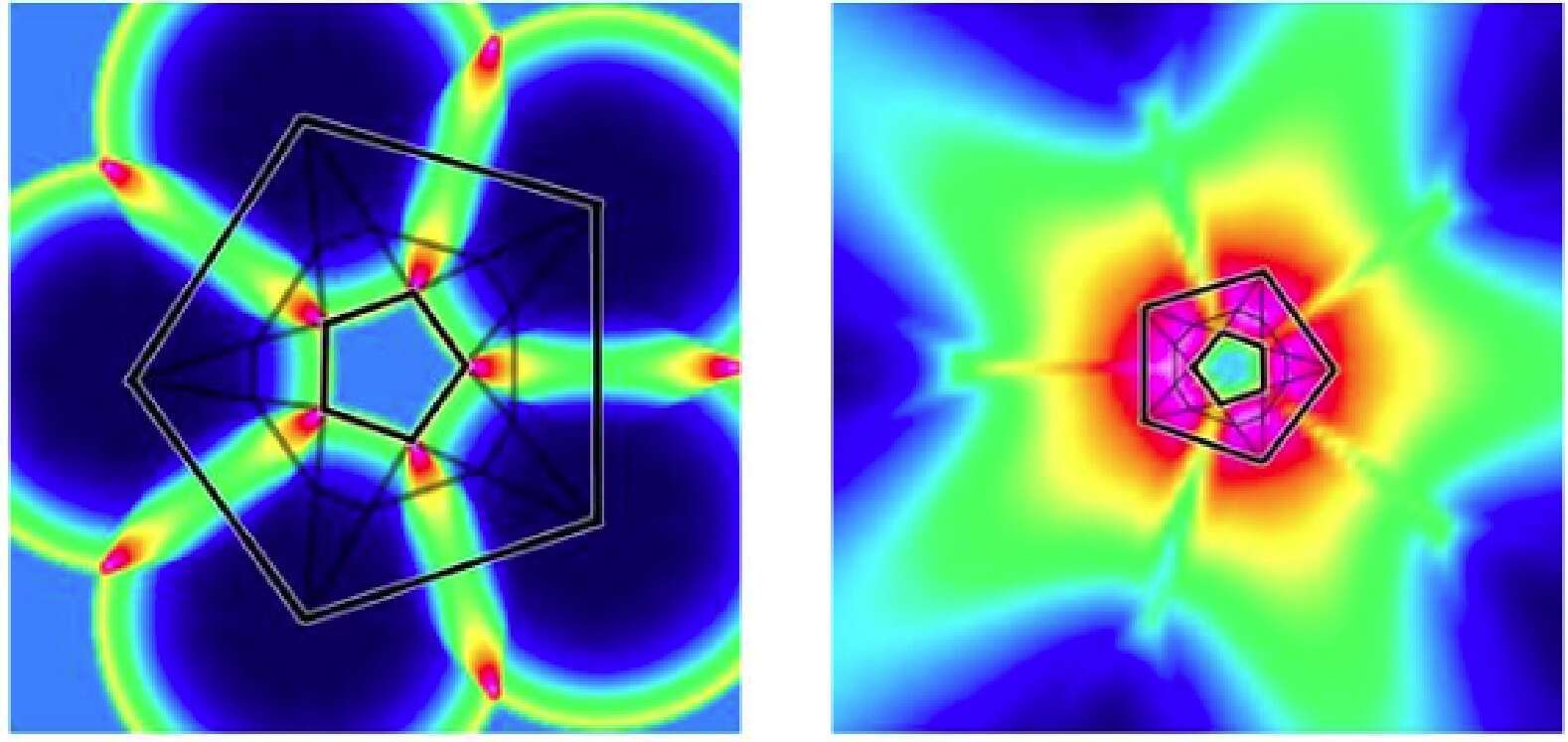

Figure 1: Polygonal reconfiguration for 5 and 10 munitions
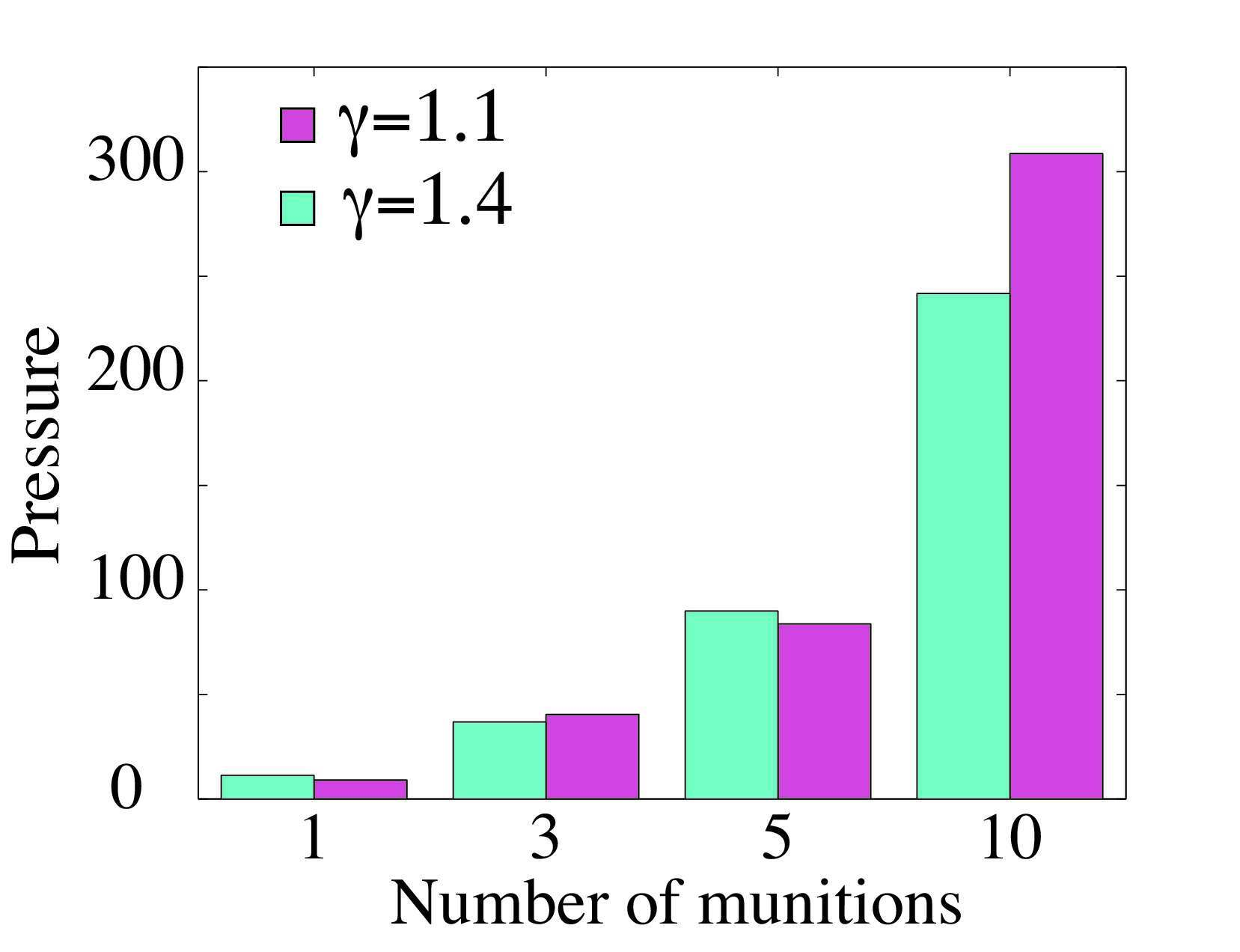
Figure 2: Non-dimensional peak pressure at target for 1, 3, 5, and 10 munitions for two different adiabatic indices
References
[1] Taylor, Geoffrey. ”The formation of a blast wave by a very intense explosion. I. Theoretical discussion.” Proceedings of the Royal Society of London. Series A, Mathematical and Physical Sciences (1950): 159-174.
[2] Schwendeman, D. W., and G. B. Whitham. ”On converging shock waves. ”Proceedings of the Royal Society of London. A. Mathematical and Physical Sciences 413.1845 (1987): 297-311.

