A bow shock always appears in front of a blunt body placed in steady supersonic flows. The stand-off distance of the detached bow shock represents, according to the existing steady flow theory, the Mach number of the steady supersonic flows (Ref. 1). We became interested in the visualization of shock stand-off distance at the transonic flow regime, particularly at very close to sonic speed, firstly in a ballistic range and secondly in shock tube flows. Convincing that, in principle, sonic flows were considered to be readily obtainable in shock tube flows, we tried to visualize flows at around sonic speed over a 20 mm diameter sphere installed in a 100 mm ×180 mm shock tube. Then we realized that it was very difficult to achieve consistently sonic flows in the shock tube. No wind tunnels are known to have been operated at exactly sonic speed. As far as we could find in the archived literature, there were no shock stand-off distance images over blunt bodies at steady near-sonic flow.

Fig. 1: Detached Ms = 0.998, Re = 9.20 ×105
We then launched in a ballistic range facility, 40 mm diameter nylon spheres into quiescent air and visualized the bow shock by double exposure holographic interferometry. The shock stand-off distances so far collected agreed well with previously published results (Ref. 1). However, we did observe a bow shock in front of the sphere in subsonic flows as well as shown in Fig. 1. Repeating the ballistic range experiments by launching 10 mm diameter spheres, we visualized the flow by high-speed imaging using a high-speed video camera. Figure 2 shows a summary of the experiments, as the relationship between the shock stand-off distance and the Mach number of the projectiles (Ref.2). Bow shocks do appear in front of subsonic blunt bodies in unsteady flows, where the shock stand-off distance diverges as the projectile speed decelerates to lower subsonic speed.
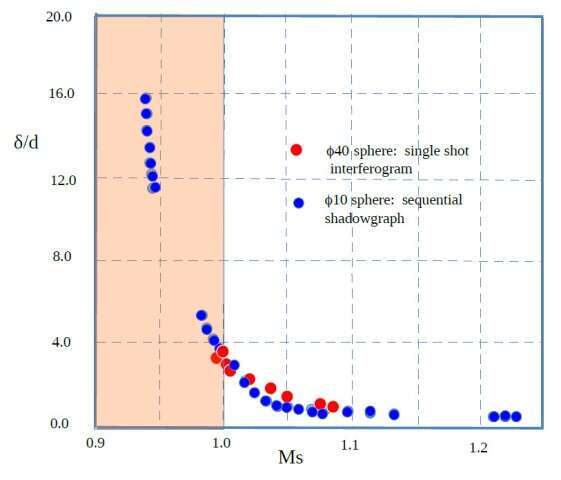
Fig. 2: Shock stand-off distance and Mach number of waves: red filled circles show interferometric data; b blue filled circles show data out of sequential images
We, shock wave researchers, are familiar with one of its extreme cases analogous to Fig.2. It is the shock tube flows, in which a contact surface is equivalent to the projectile, and a bow shock is equivalent to the shock wave driven ahead of the contact surface in a fashion analogous to a moving piston. Another example is observable in high-speed train tunnel sonic boom scenarios, where in a long tunnel a high speed train running at 100 m/s drives ahead a weak shock wave of Ms = 1.003. We then suggest, as our tentative understanding concerning bow shock stand-off phenomena, that the abscissa of the figure for shock stand-off distance versus flow Mach number in Ref. 1, may well be uniquely related to the (slightly supersonic) shock Mach number.
[1] H.W. Liepmann and A Roshko: Elements of Gasdynamics, John Wiley and Sons Inc 1960
[2] K. Takayama et al.: Shock Stand-off Distance over Blunt Bodies Projected at Supersonic Speed into Air, Water and Sand Layer, Presented at ISIS21 Riga (2014)

