The study has been conducted to examine the new methods of detonation initiation and to analyze the possible use of solutions of 2D non-stationary flows of combustible mixture to evaluate the stationary supersonic flows in 3D channels of variable cross-section. The results of numerical simulation of detonation in flat chambers with movable walls and detonation in 3D channels special dependence, blown by supersonic flows of combustible mixture, are presented and compared. The study is carried out in a framework of one-step combustion kinetics by numerical method based on the S.K. Godunov scheme.
The flows in a contracting square chambers filled with air were studied for different constant values of square sides velocity. Complicated wave patterns of the flows were obtained (Fig. 1).
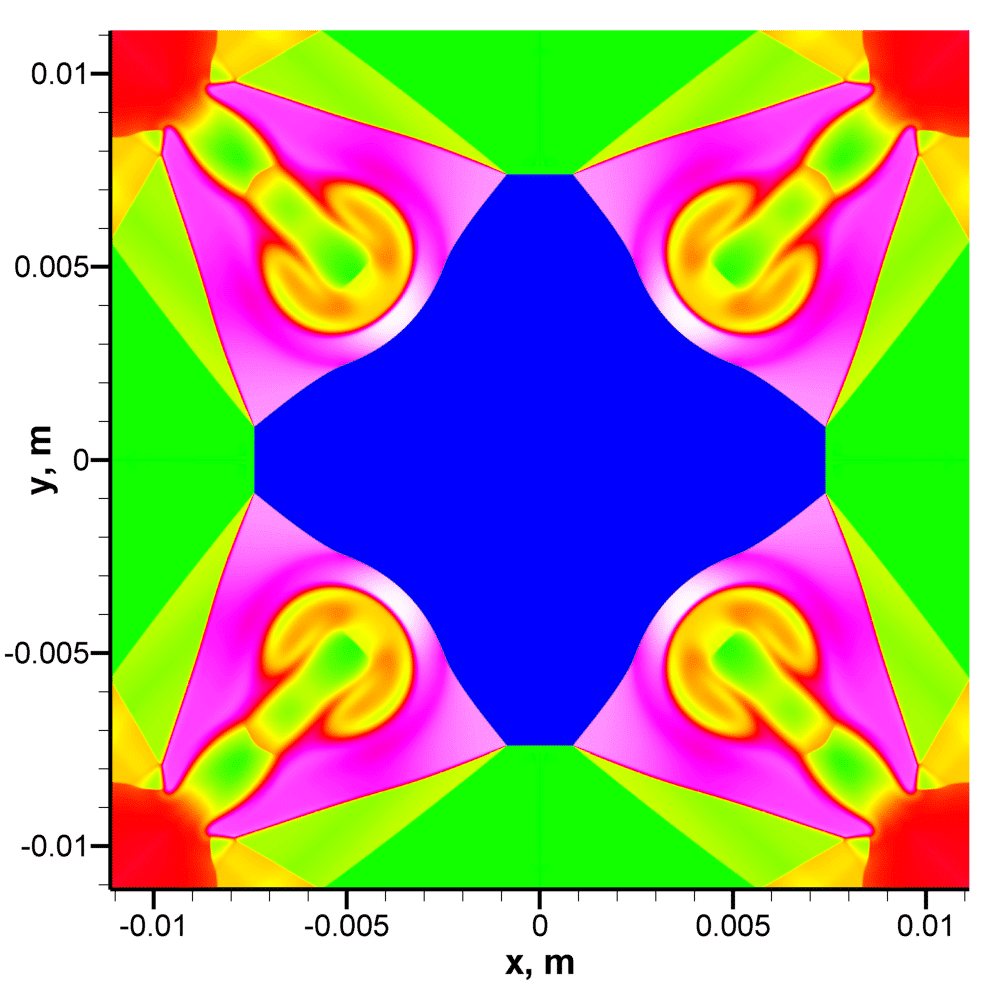
Fig. 1. Temperature field in air inside the contracting square chamber
The problem of detonation initiation in a square region with sinusoidally changing length of the side was studied. In order to identify all possible flow regimes, 196 simulations for different pairs of oscillation amplitude and period have been performed. In numerical simulations detonation could occur instantly at the 1st period along the whole perimeter of the square or at the 1st period in the corners. Initiation of detonation can take place after time interval at the center at the 1st period or after several periods. As calculations showed, in the latter case detonation may occur also on the sides, in the corners or at the center. The calculations revealed flow regimes with initiation of detonation only after 6 periods of square size oscillations. All possible flow regimes are divided in a plane “amplitude-period” by critical curves.
The problem of formation of detonation in three-dimensional channel with square variable cross-section was considered. Detailed stationary (Fig. 2) and unsteady flow patterns with and without detonation have been found. The critical values of gas-dynamic parameters separating different flow regimes were obtained. Hypersonic analogy of flat and three-dimensional flows, allowing the use of two-dimensional solutions to estimate the parameters of three-dimensional supersonic flows, has been confirmed. The limitations of the analogy have been determined, the main of which is the chocking effect of the burning flow (Fig. 3).
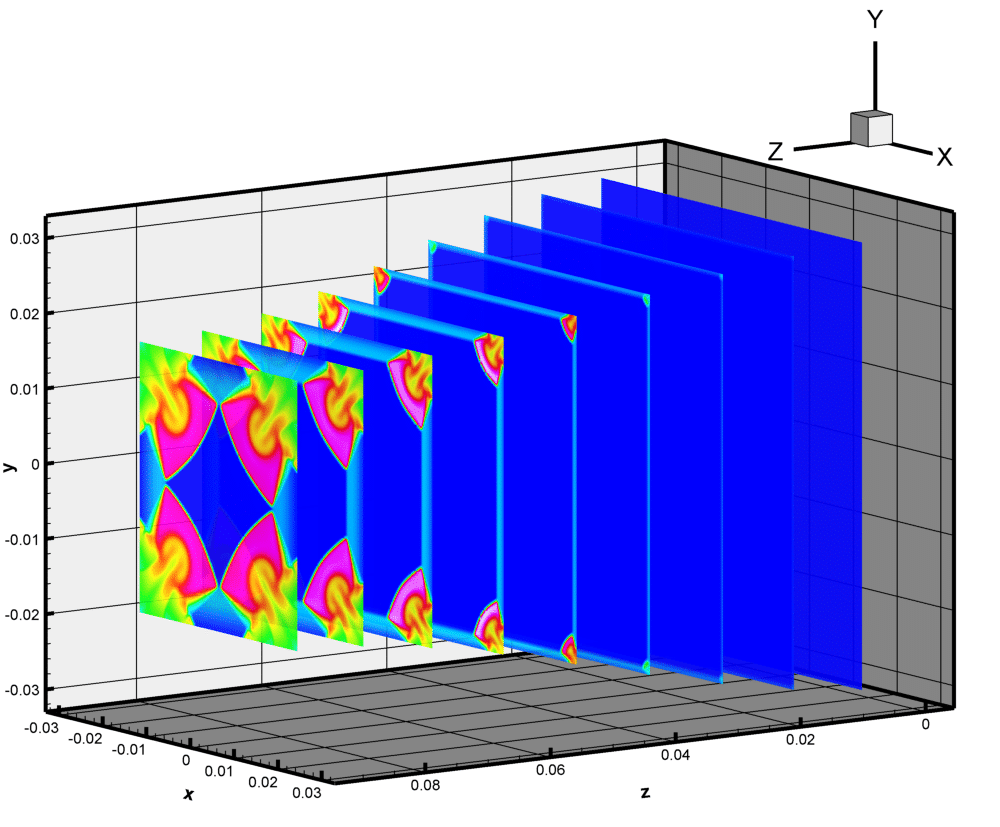
Fig. 2. Temperature field slices in the case of stationary detonation of supersonic combustible mixture flow in 3D channel
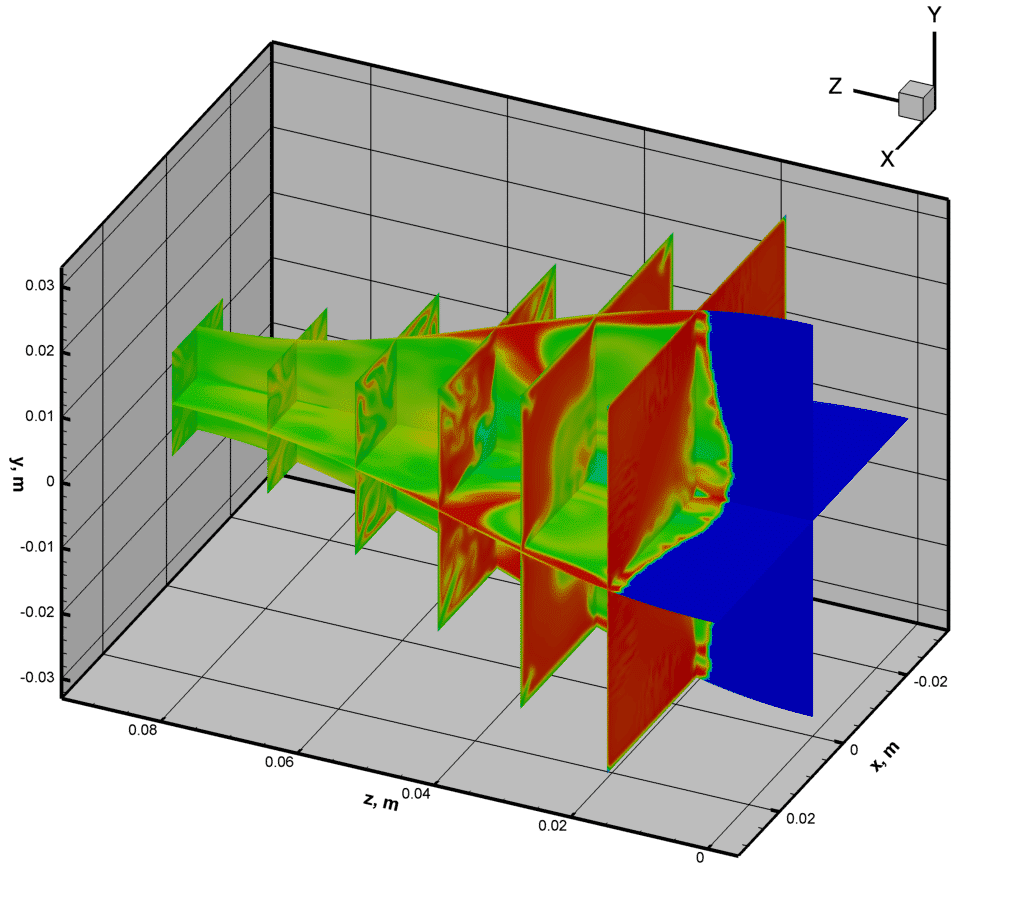
Fig. 3. Temperature field slices for detonation in supersonic combustible mixture flow with choking effect and inapplicability of hypersonic analogy
Detonation initiation using rotation of the elliptic cylinder enclosed in the circular cylinder, both filled with stoichiometric air-propane mixture, was numerically investigated. The feasibility to form detonation both inside, and outside the elliptic cylinder was stated. Two critical angle velocities of cylinder rotation, which govern the quantitative and qualitative flow pattern, were found. The possibility of rapid detonation initiation by rotating parabolic blades was studied in two configurations (Fig. 4a, 4b).
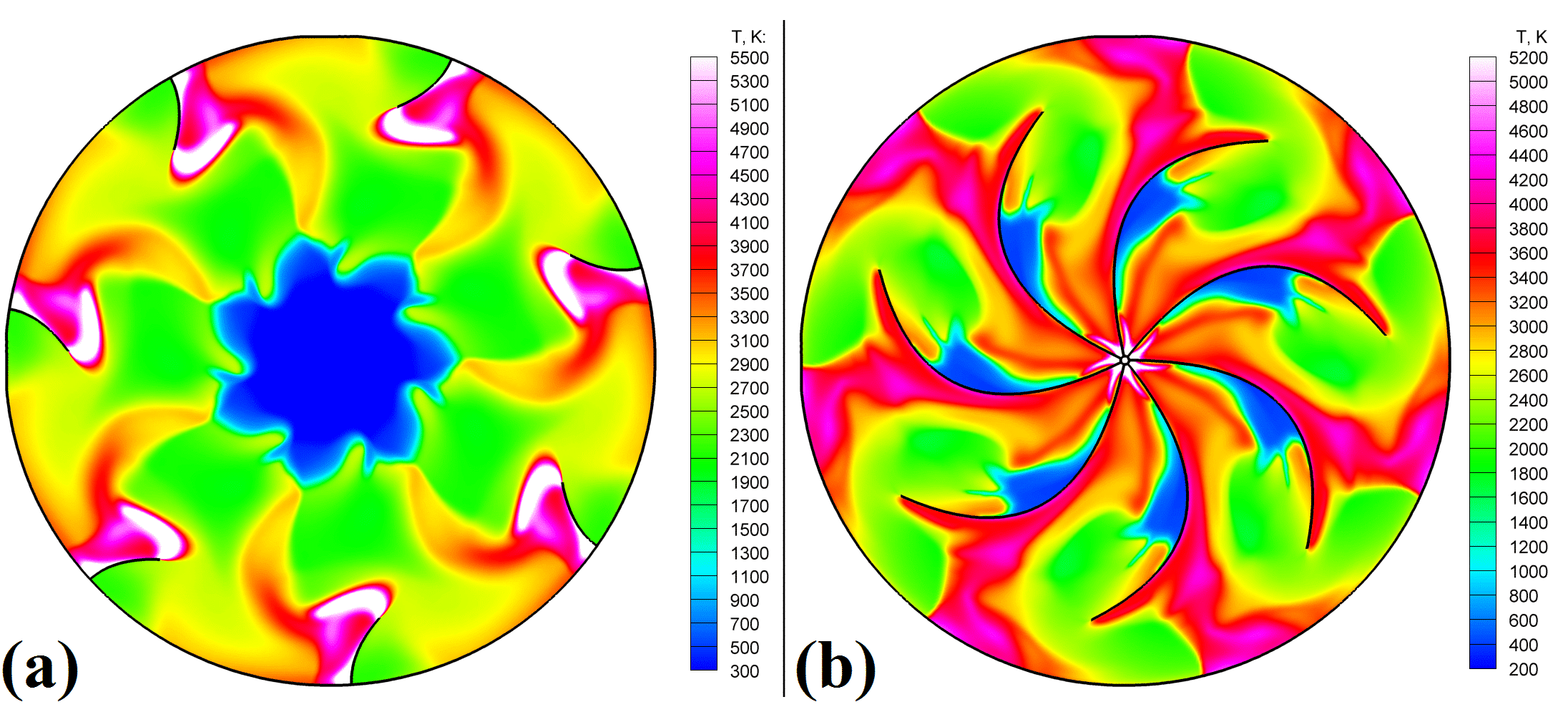
Fig. 4. Formation of detonation inside the rotating cylinder with parabolic blades (a) and inside the cylinder with rotating star in the center (b)
The method to estimate parameters of the supersonic flows in three-dimensional spiral channels is proposed based on the plane-sections hypothesis. The comparison with 3D simulation is presented (Fig. 5, 6, 7).
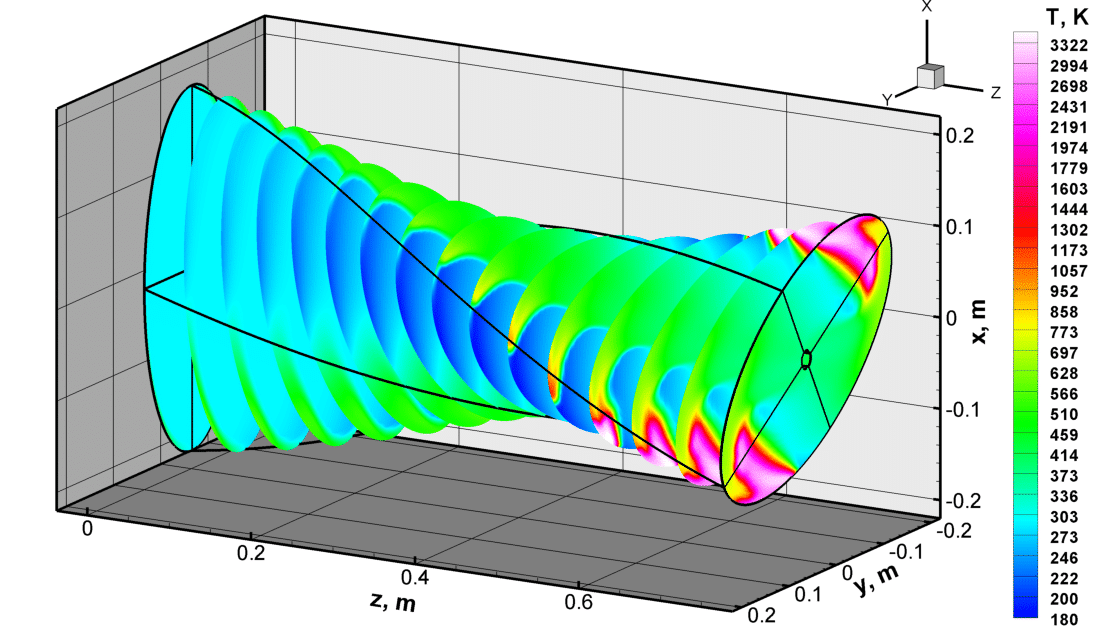
Fig. 5. Slices of stationary temperature field under detonation in helical channel of elliptic cross-section
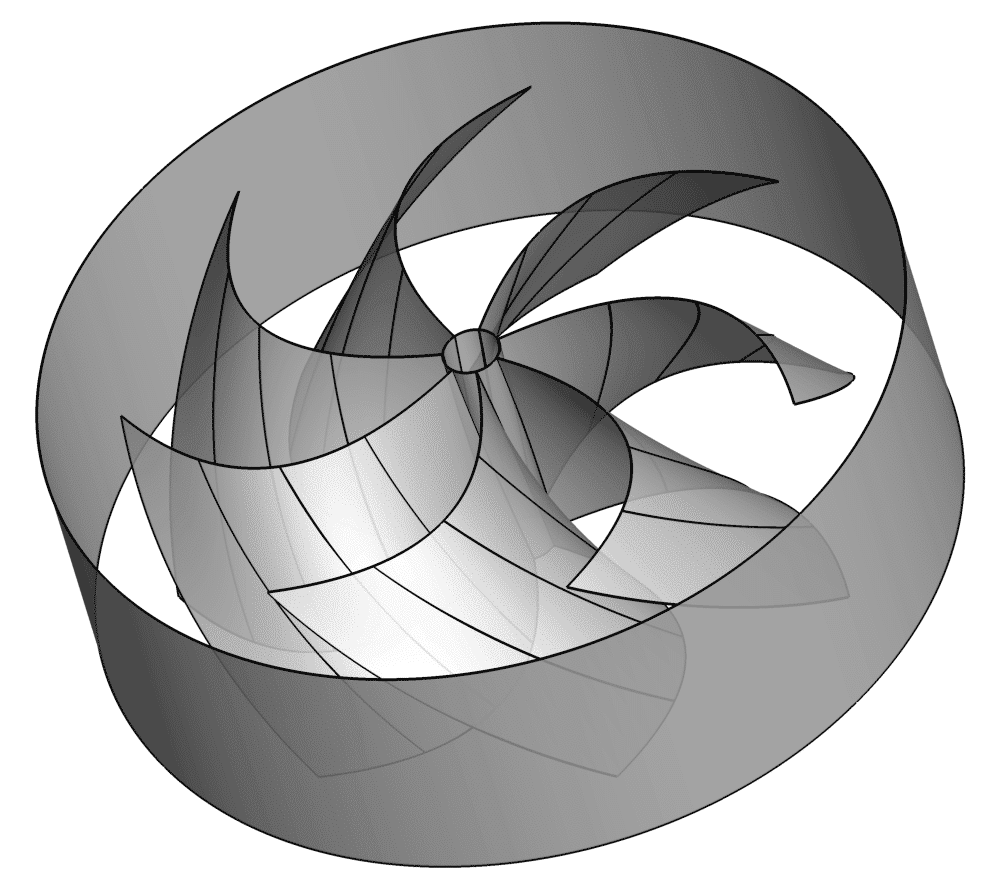
Fig. 6. Geometry of the channel with blades
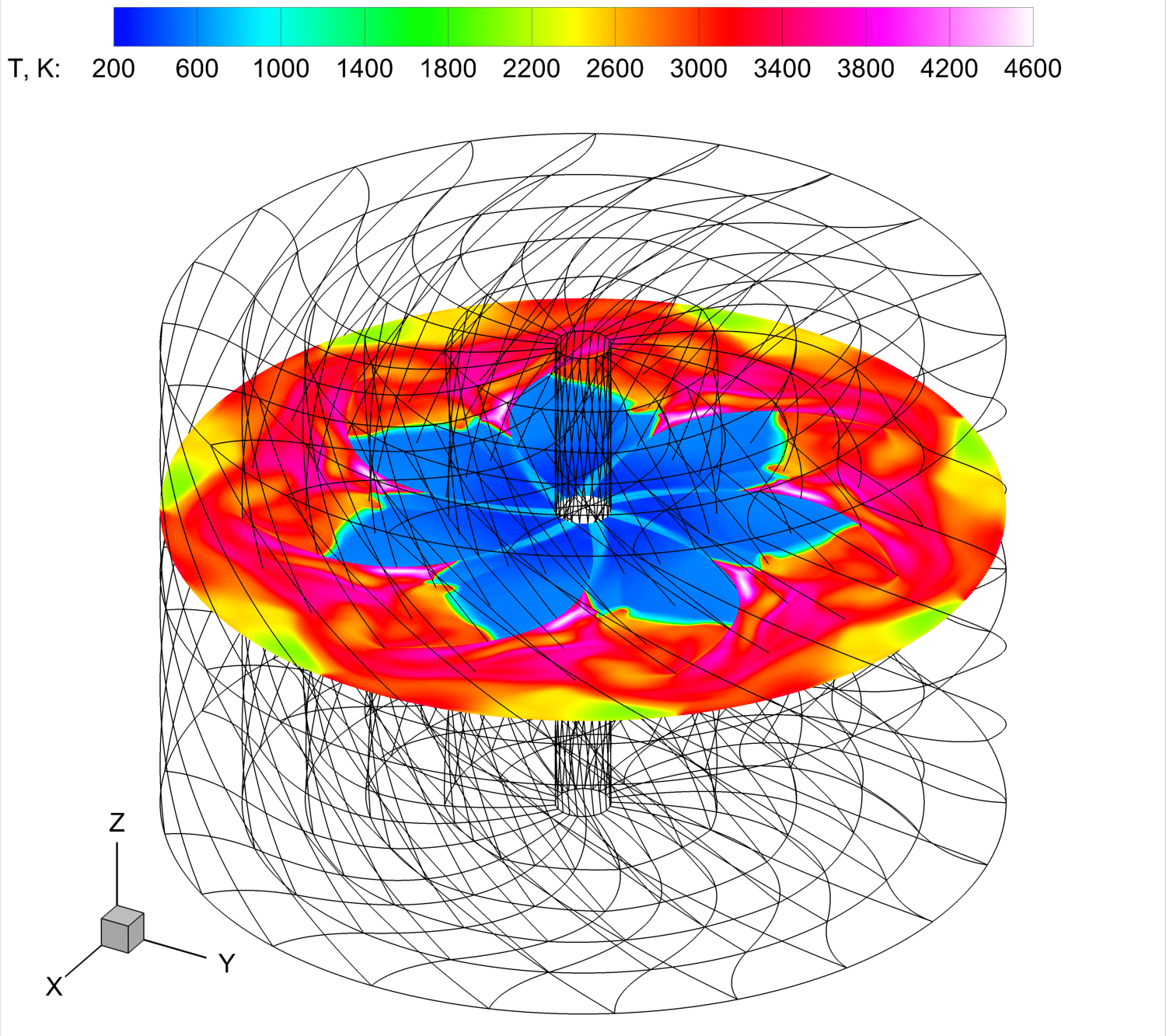
Fig. 7. A slice of stationary temperature field with detonation in channel with blades
The original parallelized software package with graphical interface was developed and used to perform numerical investigation. The calculations were performed on supercomputer “Lomonosov”.
The work was supported by the Russian Science Fund (Grant No. 14-19-01759).

