The problem of shock-vortex interaction in the case when both the velocity of the shock wave and velocity in the vortex are comparable with the speed of light is investigated numerically on the basis of special relativistic hydrodynamics equations [1] in two-dimensional formulation. The problem is formulated in the frame attached to the shock wave front. The equation of state p=(γ-1)(e-ρc2) is used, where γ=const, p and e are the pressure and the energy density in the rest coordinate system, ρ is the rest mass density. The Lorentz contraction of the vortex in the direction of motion, which takes place in this frame, is found to be challenging for numerical resolution of shock-vortex interaction. In the present work a high resolution numerical method based on the approximate Riemann solver [2] has been applied. Influence of shock wave Lorentz factor on the solution is investigated. As in the case of classical hydrodynamics the interaction generates secondary shocks, which propagate along the shock wave front. It is found that the sound generation decreases with increasing of the shock wave Lorentz factor. An example of complicated flow in which vortices interact with internal shock waves of the relativistic jet is presented (Figure 1).
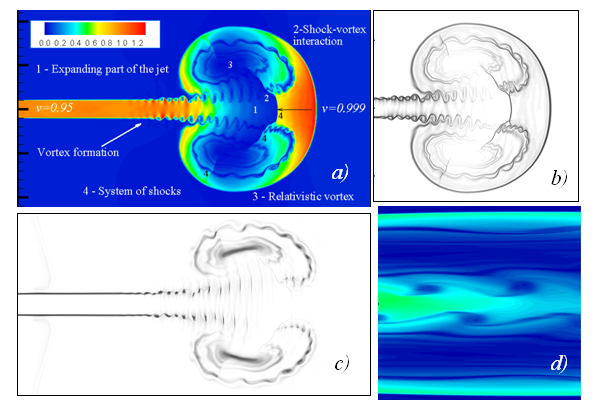
Figure 1 (a) the rest mass density; (b) absolute value of the density gradient; (c) the absolute value of vorticity; (d) the rest mass density in the frame moving with the vortices.
The jet is injected into the half-space, which contains a matter being at rest in the coordinate system attached to the boundary. The conditions correspond to ‘naked’ jet with high internal energy both in jet and in the surrounding medium (the morphology of relativistic jets with different properties has been studied numerically for two decades, see review [3]). The jet instability [4] leads to the vortex system formation. The vortices are accelerated in the expanding supersonic part of the jet up to high magnitude of Lorentz factor and interact with the shock wave in which the jet is decelerated. In figure 1 the flowfied is presented. The rest mass density is shown in panel (a). The shock position can be seen in panel (b) where the absolute value or the rest mass density is shown; the vorticity is presented in panel (c).
[1] L.D. Landau, E.M. Lifshitz (1959). Fluid Mechanics. Vol. 6 (1st ed.). Pergamon Press
[2] Mignone A. and Bodo G. (2005) Mon. Not. R. Astron. Soc. 364, 126–136
[3] Marti J.-.M., Muller E. (2003) Living Rev. Rel. 6: 7
[4] Marti J.-M., Perucho M. (2004) Astrophysics and Space Science, 293, Issue 1-2, 139-147

