Magnetic resonance force microscopy(MRFM) is an imaging technique that enables acquisition of three-dimensional magnetic images at nanometer scales, and has been adapted for the detection of magnetic spin of a single electron. It is based on combining the technologies of magnetic resonance imaging(MRI) with atomic force microscopy(AFM). Recently, MRFM techniques have been used to estimate dynamic nuclear polarization in addition to detection of electron spin magnetization. In conventional MRI devices the electronic spins are detected by measuring their magnetic induction using an inductive coil as an antenna. However, in MRFM the detection is implemented mechanically using a cantilever to directly detect a modulated spin gradient force between the sample spins and a ferromagnetic particle attached to the cantilever tip(Fig.1a). While MRFM systems are receiving a growing amount of interest, to date, a comprehensive theoretical treatment is still lacking. Existing models are based on simplistic lumped-mass reductions that include linear estimates of cantilever stiffness and damping complemented by a nonlinear approximation of the magnetic force. We thus consistently formulate a nonlinear initial-boundary-value problem (IBVP) combining the micro-cantilever motion and the magnetic moments of the spin. We reduce the IBVP to a nonlinear dynamical system and investigate stability of the equilibrium solution of the respective autonomous system for different values of a magnetization parameter to reveal both pitchfork and saddle-node bifurcations(Fig.1b). Numerical integration of the dynamical system and computation of the response Poincare’ maps reveal existence of lengthy chaotic-like transients for low damping near primary resonance(Fig.1c).
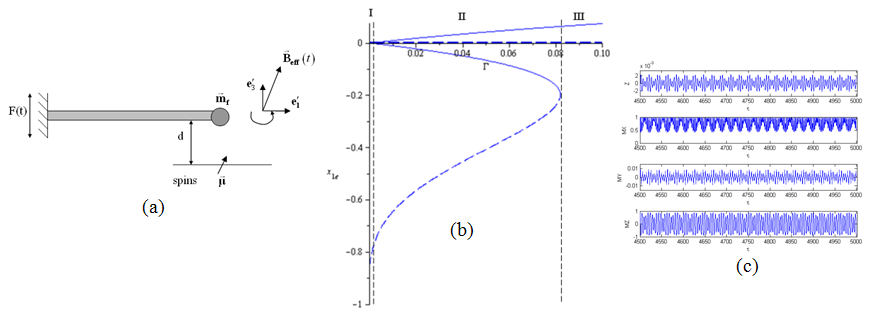
Figure 1:(a)Definition sketch of the MRFM setup. (b)Beam displacement equilibrium against normalized magnetization parameter depicting pitchfork and saddle-node bifurcation structures. (c)Time series of beam displacement and magnetic moment components of a chaotic transient solution.

