We discuss an implementation of immersed boundary method (IBM) for analysis of convective and diffusive heat transfer problems. Direct forcing approach with auxiliary correction step is utilized to enforce Dirichlet boundary conditions for both velocity and temperature fields. We show that the method can generically be embedded into any projection approach based Navier-Stokes time stepper, resulting in a minimal amount of changes required. An implemented approach has been efficiently used for investigation of a natural convection flow inside differentially heated spherical annuli. A number of spherical annuli configurations with embedded internal walls of different geometries and orientations are simulated. A parametric study aimed at investigation of typical flow patterns and increasing insulating properties of the spherical annuli is performed.
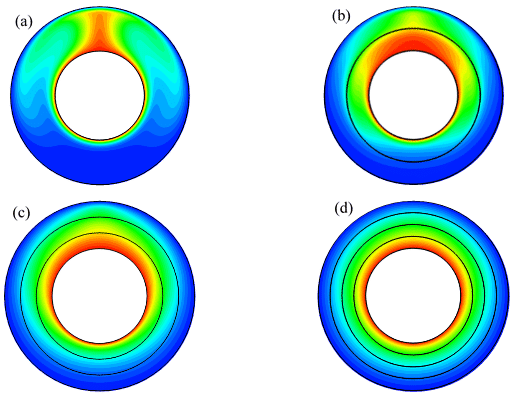
Figure Temperature distribution inside differentially heated spherical annuli, with Ri/Ro=0.5, Ra=105: (a) no internal walls ; (b) one internal wall; (c) two internal walls; (d) three internal walls.

