It is well-established experimentally and by direct numerical simulations that a pulsatile flow in a stenotic tube can undergo through transitional and intermittent laminar-turbulent states. Even at relatively low Reynolds numbers, the high degree of occlusion creates a jet flow, which at certain (instantaneous) value of the Reynolds number becomes unstable, leading to onset of turbulence. In particular, the turbulent regime starts downstream of the stenosis extending about four to six tube diameters further downstream. As the Reynolds number decreases, flow re-laminarization takes place. Since the standard RANS-based models of turbulence are derived for fully developed high Reynolds number turbulence, they are not appropriate for modeling transitional flows. Nevertheless, our results show that, being properly applied, model of turbulence reasonably predicts transient flows in a stenotic tube. We formulated the computational setup requirements and procedure for simulating laminar-turbulent transient flows by turbulence transport models. The boundary conditions and the computational domain length were chosen to meet the basic constraint of the laminar-turbulent-laminar transition. In particular, it means that the flow is laminar upstream of stenosis, becomes turbulent immediately after it and reverts to laminar downstream in the recovery region. The measure for turbulence used in this study is the so-called turbulent viscosity ratio (TVR=) defined as the ratio between the turbulent and kinematic viscosity. We note that small values of TVR signify insignificant turbulence. Figure presents TVR at the peak systolic instance for a case with a radial stenosis S=85%. It is evident that the flow enters the tube as laminar, becomes turbulent upon passing the stenosis and recovers downstream back to laminar.
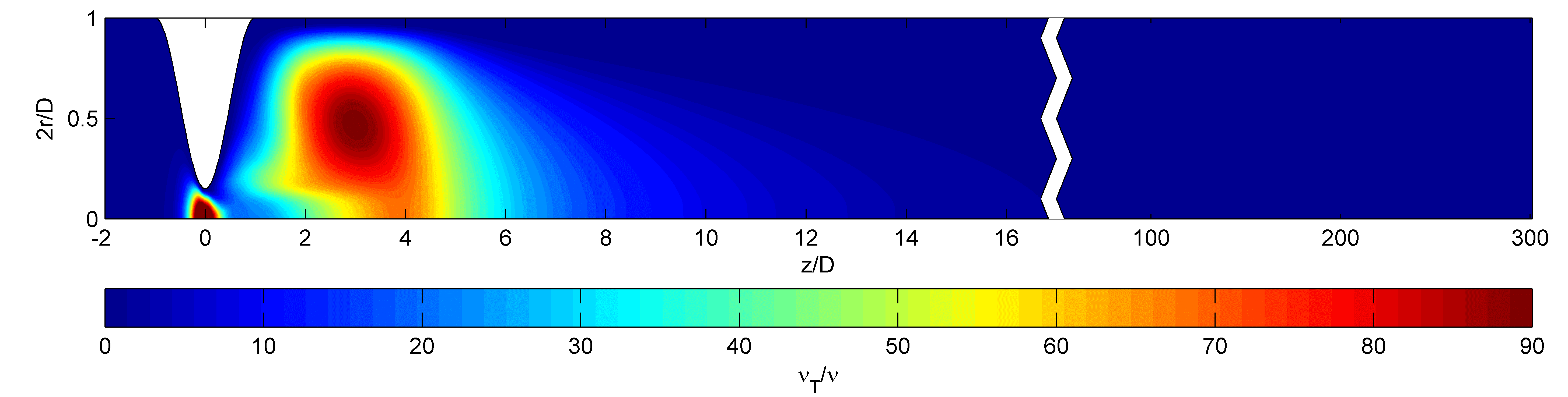
We have performed comparative analysis of flow patterns and characteristics for a wide range of stenoses. We demonstrated that for severe stenoses, a pulsatile flow exhibits the features of a quasi-steady flow.

