Effects of Faceting in the Optical Response of Plasmonic Gap Nanoantennas and Chains

Garikoitz Aguirregabiria Theory of Nanophotonics, Cenatro de Física de Materiales, Centro Mixto CSIC-UPV/EHU and Donostia International Physics Center (DIPC), Donostia-San Sebasián, Spain Ruben Esteban Theory of Nanophotonics, Cenatro de Física de Materiales, Centro Mixto CSIC-UPV/EHU and Donostia International Physics Center (DIPC), Donostia-San Sebasián, Spain Andrei G. Borissov Institut des Sciences Moléculaires d'Orsay, UMR 8214, CNRS-Université Paris-Sud, Orsay, France Yumin M. Wang Departament of Electrical and Computer Engineering, MS378, Laboratory of Nanophotonics, Rice University, Huston, Texas, USA Peter Nordlander Departament of Electrical and Computer Engineering, MS378, Laboratory of Nanophotonics, Rice University, Huston, Texas, USA Garnett W. Bryant Joint Quantum Institute and Quantum Measurement Division, National Institute of Standards and Technology, Gaithersburg, Maryland, USA Javier Aizpurua Theory of Nanophotonics, Cenatro de Física de Materiales, Centro Mixto CSIC-UPV/EHU and Donostia International Physics Center (DIPC), Donostia-San Sebasián, Spain

Plasmonic gap-nanoantennas are suitable platforms for applications in field-enhanced spectroscopies and sensing due to the strong near-field localization and enhancement that they produce. Plasmonic gaps show distinctive resonances that can be tuned by a convenient manipulation of the gap load or morphology[1,2]. In this work we study how critically the exact faceting of the gap affects the optical response of antennas formed by metallic nanorods. We first report the plasmonic response of antennas with spherical gap termination, that resemble the behavior found for nanosphere dimers[3], showing plasmonic modes that exponentially redshift as the antenna arms are approached and diverge at contact. However, as the gap morphology becomes flatter, the dispersion of the longitudinal antenna plasmon (LAP) saturates for very narrow gaps (fig.1a)[1]. Furthermore we observe a new set of very narrow and strongly redshifting transverse cavity plasmons (TCP) that appear in the near field spectrum as Fabry-Pérot cavity modes (fig.1a). For subnanometric gaps we study how electron tunneling affects the optical response finding drastic differences between spherical and flat gaps. Moreover, when plasmonic gaps are chained, capacitive chain plasmons (CCP) are formed which can be tuned by controlling the number of gaps in the chain[2]. We also study how the faceting of this gaps affects the tunability of the CCPs. We find that as the facet size increases, the shift in the CCP as a function of chain length becomes larger, allowing for a better tunability of the resonance than with spherical particles(fig.2). Faceted gaps are promising structures for controlling photochemistry and optoelectronic processes.

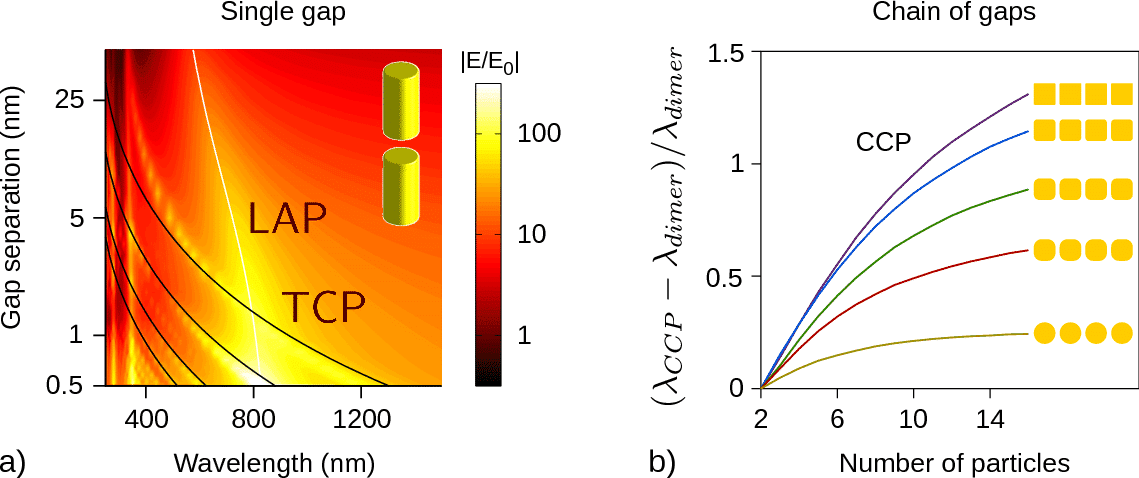
Figure: (a) Near-field enhancement spectrum as a function of wavelength and gap separation of a flat-gap antenna showing the dispersion of the longitudinal antenna plasmon (LAP) and transverse cavity plasmons (TCP). (b) Relative shift of the capacitive chain plasmon (CCP) as a function of the number of particles in the chain for different faceted particles.

[1] Esteban. R., et al, ACS Photonics.

[2] Herrmann, L.O., et al, Nature Comm., 5, 4568 (2014).

[3] Romero, I., et al, Optics Express, 14, 9988 (2006).

garikoitz_aguirregabiria001@ehu.es