Mapping and Interpreting the Near Fields of Plasmonic Antennas

Tomáš Neuman Material Physics Center, Spanish National Research Council and University of the Basque Country, San Sebastián, Spain Donostia International Physics Center, DIPC, San Sebastián, Spain Central European Institute of Technology, Brno University of Technology, Brno, Czech Republic Pablo Alonso-González Nanophotonics Laboratory, CIC nanoGUNE, San Sebastián, Spain Aitzol Garcia-Etxarri Material Physics Center, Spanish National Research Council and University of the Basque Country, San Sebastián, Spain Donostia International Physics Center, DIPC, San Sebastián, Spain Pablo Albella Material Physics Center, Spanish National Research Council and University of the Basque Country, San Sebastián, Spain Donostia International Physics Center, DIPC, San Sebastián, Spain Rainer Hillenbrand Nanophotonics Laboratory, CIC nanoGUNE, San Sebastián, Spain Javier Aizpurua Material Physics Center, Spanish National Research Council and University of the Basque Country, San Sebastián, Spain Donostia International Physics Center, DIPC, San Sebastián, Spain

Plasmonic antennas concentrate and enhance electromagnetic fields into regions below diffraction limit. Advanced theoretical and experimental techniques are necessary to fully characterize the antenna near fields [1], as in scattering-type Scanning Near-field Optical Microscopy (s-SNOM), accessing both the near-field amplitude and phase. The cross-polarized detection scheme utilized in the s-SNOM measurements enables to distinguish the signals recorded in S- and P-polarizations [2]. Here we review and modify the common assignment of the S- and P-resolved signals to the in-plane and out-of-plane components of the local near field around the antenna, respectively.

We interpret the s-SNOM signal obtained with use of weakly scattering tips as a scalar product of the real antenna electric near field and a virtual electric field which would be induced in the antenna by a source placed at the position of the detector with the same polarization as the detected light. We demonstrate theoretically and experimentally that the s-SNOM near-field signal measured on single and dimer plasmonic antennas can be understood as a result of a mixing of the antenna electric near-field components rather than measurement of the in-plane or out-of -plane electric fields.

The novel signal interpretation of s-SNOM images verifies a quadratic dependence of the signal amplitude on the antenna near-field enhancement resembling the enhancement mechanism in field enhanced spectroscopies [3]. S-SNOM is an ideal tool for local characterization of the antenna near-fields, but as shown here, an appropriate interpretation of the signals is required for a correct understanding of the images.

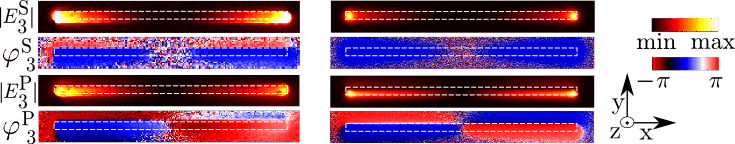
Experimental (left) and theoretical (right) near-field maps of a linear dipole antenna. The antenna length is 3.2 m and the probing wavelength is =11.06 m.

Fig. 1: Experimental (left) and theoretical (right) near-field maps of a linear dipole antenna. The antenna length is 3.2 µm and the probing wavelength is λ=11.06 µm.

[1] P. Alonso-González et al., Nano Lett. 11, 3922 (2011).

[2] M. Schnell et al., Nano Lett. 10, 3524 (2010).

[3] P. Alonso-González et al., Nature Comm. 3, 684 (2012).

[4] T. Neuman et al., (submitted)

tomas_neuman001@ehu.es