
Three-Wave-Mixing in Nonlinear Metamaterial Photonic Crystals
Non-linear photonic crystals enable control over quadratic nonlinear optical interactions [1] and allow to create functional optical devices e.g. frequency converters, switches, beam shapers and optical amplifiers. However the miniaturization of these devices is limited by the properties and ease of integration of the nonlinear crystals available in nature. Recently a new family of nanostructured plasmonic optical materials, so-called metamaterials (MM), with artificial effective tunable nonlinearities, were demonstrated [2]. Controlling their nonlinear output can open a whole new area for potential fundamental research and development of efficient, active, integrated and ultra-compact nonlinear optical devices.
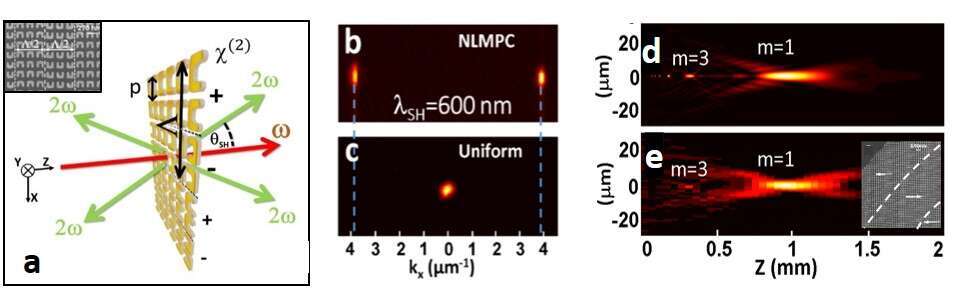
Here we demonstrate experimentally exceptional control of the nonlinear emission from a new family of nonlinear metamaterials which form nonlinear, metamaterial-based, photonic crystals. We construct the nonlinear MM from plasmonic split ring resonators, and modulate their quadratic nonlinearity in different regions by geometric manipulations which impose π phase shift on the locally generated nonlinear signal (Fig. 1a). This modulation mimics domain inversion in conventional nonlinear photonic crystals. We use it to demonstrate engineered nonlinear one-dimensional (Fig. 1b and c) and two-dimensional diffraction and all-optical scanning, enabling ultra-wide angular scan of the nonlinear output from the MM. In addition we demonstrate intense focusing of the nonlinear signal directly from the MM by designing a non-linear Fresnel Zone plate (Fig. 1d and e), resulting in nearly two orders of magnitude enhanced intensity [3].
In the meeting we will present also a theoretical study of the nonlinear dynamics in three-dimensional structures formed from stacked multiple MM layers. We will show that this enables giant enhancement of the total conversion efficiency and examine the effects of perfect and quasi phase matching in these structures. Finally we will also present an extension of the process of second harmonic generation in these artificial structures to three-wave-mixing processes in general e.g. sum- and difference-frequency generation and discuss their properties.
[1] V. Berger, Phys. Rev. Lett. 81, 4136 (1998).
[2] M. Kauranen and A. V. Zayats, Nat. Photonics 6, 737–748 (2012).
[3] N. Segal, S. Keren-Zur, N. Hendler, T. Ellenbogen, Nat. Photonics , to be published (2015).
shaykerenzur@post.tau.ac.il
Powered by Eventact EMS