
Surface Waves on Hyperbolic Metasurface
In this work we analyze new type of surface electromagnetic waves which can propagate along hyperbolic metasurface (Fig.1a), i.e. along the plane layer which can be described by a conductivity tensor with indefinite signature (Im(σ||) Im(σ⊥)<0). We propose that σ|| and σ⊥ have Drude-Lorentz dispersion with resonant frequencies Ω|| and Ω⊥ (Fig.1b). Therefore, regime of hyperbolic metasurface is realized in the range Ω⊥<ω<Ω||. We have shown that there are two surface waves of hybrid TE-TM polarization which can propagate along the metasurface. Their dispersion for different propagation angles α is shown in Fig.1c.
Equal frequency contours, optical losses and electromagnetic field distribution are analyzed in detail. The analysis showed that the surface waves have unusual polarization properties and have common features with Dyakonov surface waves [M.I. Dyakonov, JETP 67, 717 (1988)] and magnetoplasmons [K. W. Chiu and J. J. Quinn, Phys. Rev. B 9, 4724 (1974)].
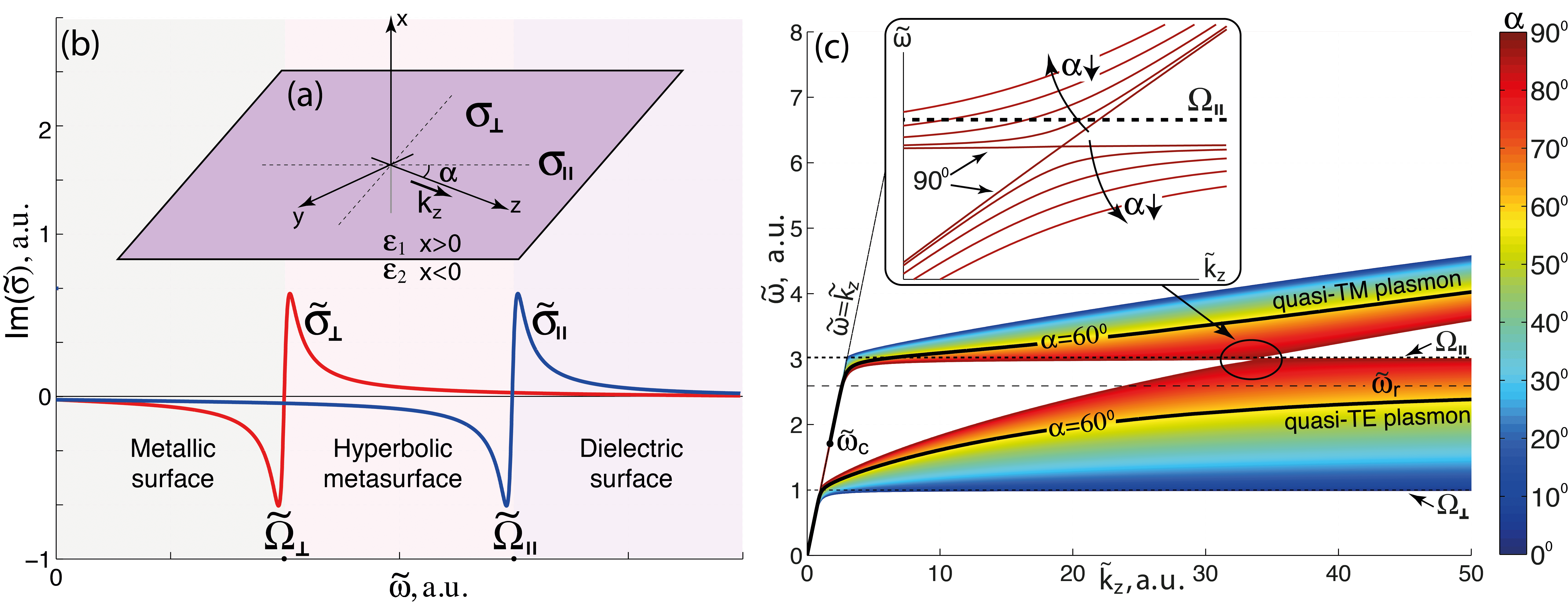
Fig.1 (a) Hyperbolic metasurface separating two dielectric media. Conductivities σ|| and σ⊥ correspond to the principle axes of the conductivity tensor. (b) Conductivities σ|| and σ⊥ have Drude-Lorentz dispersion with resonance frequencies Ω|| and Ω⊥. α is the angle between wavevector kz and principle axis of the conductivity tensor corresponding to σ||. (c) Dispersion of surface waves depending on propagation angle α.
bogdan.taurus@gmail.com
Powered by Eventact EMS